Physical Quantities and Measurement II

Hello friends,

Welcome to the exciting world of “Physical Quantities and Measurement II” These ideas are like the foundations of science, helping us measure and understand things like length, time, weight, and temperature. They make it easier for us to grasp how the physical world works and allow us to measure things accurately in science. So, let’s explore together how they’re essential in uncovering the secrets of the universe.

Measuring Length

In simple words, length means the distance of a straight line travelling through two points. To express distance through different methods, we use length, breadth (width), depth and height. The SI unit of length is metre and we denote it by an ‘m’.

To avoid confusion in the length of a metre throughout the world, the standard metre is put between two marks on a platinum-iridium bar kept at 0 degree located at the International Bureau of Weights and Measures in Sevres near Paris in France.

Every country owns a duplicate of the original metre for correct measurements of metre ruler which they manufacture. In India, the standard metre is there at the National Physical Laboratory in Delhi.

Common devices used to measure length

Some of the common devices used to measure length are –

1. Ruler –

Used for small measure.
Generally, available in 15 cm (6 inches) or 30 cm (12 inches).
A centimetre is further divided into 10 millimetres.

2. Metre Rod –

Has a fixed length of a metre.
It has 100 divisions, each of 1 cm (1m = 100cm).
A centimetre has 10 millimetres.
Generally, used by cloth merchants (tailors).

3. Measuring Tape –

Can contain more than one metres.
Used to measure long lengths (length of a field).
Have a flexible movement to measure curved objects too (size of a person’s chest, circumference of a tree trunk).

How to use a ruler correctly?

Reading correct measurement using a ruler plays a very important role. So, in order to take the correct measurement from the ruler, you will have to follow these certain steps.

• Avoid using the ends of a ruler – If you use your ruler in an improper way or you use it regularly, the edges and corners of your ruler get broken and it does not give that perfect line design on your notebook. So to prevent this problem, you should not put the left edge of the object (to measured) straight at the zero mark.

• Placing the ruler correctly – For a very correct measurement of your object, you should keep the ruler exactly parallel to the object and keep it as near as possible.

• Correct positioning of eyes – Correct positioning of eyes while measurement matters a lot. Whenever you are doing measurement with a ruler, you should always keep your eyes vertically and horizontally straight in line with the mark you are reading. Parallax error is the error which can be made while reading the measurement using a ruler.

Indirect Measurement of Length by Using a Ruler

To measure a curved or designed line using a ruler, you can do the following steps and you will definitely get the correct measurement.

Fetch a piece of thread.
Put the thread along the whole line and cut it wherever it ends.
Take a ruler and put the thread parallel to its marking.
Measure the length of the thread using the ruler.
The measured value of the curved line will be displayed.

Also read:

Measuring Mass

The amount of matter (solid, liquid, gas) present in the object is mass. The SI Unit of mass is ‘Kilogram (Kg)’. Generally, we don’t use the word mass in our daily life. Instead, we use the word weight. Suppose, if you purchase 3 kg of potatoes from a vegetable vendor then actually you are purchasing 3 kg mass of the potatoes.

The very common submultiples of mass are gram (g) and milligram (mg). We use these submultiples of mass to measure smaller quantities whereas, and quintal and metric ton to measure very big quantities.

Devices Used to Measure Mass

People have developed several devices to measure mass till date. Beam Balance/Grocer’s Balance, Electronic Weighing Machine and Physical Balance are some of the common devices for the same. Let’s move into deep detailed version of these devices.

1. Beam Balance (tarazu)

This is the simplest type of instrument which we can use to measure mass. Let’s see the structure of the beam balance:

We take the standard weights as a fixed quantity and the compare the unknown quantity with it.
Beam balance has a straight metal beam which is generally of iron.
Iron rod is supported with an iron loop in the centre.
There is a pointer between the iron loop.
We hang two identical pans from the two ends of the rod equally.
Both the pans are equal in their length and mass so that we don’t get a wrong weight.
Object (whose mass has to be calculated) is generally put on the right pan.
After that, we put the standard weights on the left pan.
Then, we put these fixed units in an increasing way on the pan till the metallic beam turns horizontal and the pointer becomes vertical.
At last, we take out the sum of the standard weights and calculate the mass of the object.

*One thing in notice that, when both the pans are empty, the metallic beam should be horizontal and the pointer should be vertical.

2. Common type of beam balance

Nowadays in the shops, there is a different type of beam balance (somewhat type of a digital machine, but not exactly digital) which is similar to the normal one. There are some changes as given in the points below-

No beam is present but a pointer is there.
The pointer moves whether right side or the left side in a rainbow shape according to the weight put on it.
So, if we compare this version with the traditional beam balance then we can say that if the pointer becomes vertical in this digital version then it means that both the pans have equal mass.

3. Electronic Weighing Machine

An electronic weighing machine is also a good device for measurement of mass. In this device, the measurement of mass doesn’t need to have the standard weights. Instead, it uses the LCD display panel right on its top which displays the mass of the object digitally.

4. Physical Balance

In the science laboratories or in a jewellery shop, the accuracy plays a very very important role. We can’t do these types of measurements using devices like beam balance. So, for that reason, we have a physical balance. A physical balance can do very sensitive calculations of mass measurement.

Measuring Time (Physical Quantities & Measurement II)

Time is very important for every task we do in our day to day life. The SI Unit of time is ‘second (s)’. Some other very common units of time that we use daily in our life is – minute (min), hour (h) and mean solar day.

Mean solar day is the time that the earth takes to complete one whole rotation in its own axis.

To measure the time of the day we use some units like- hour, minute and second. Commonly, we use the 12-hour clock system in which the day contains two periods of 12-hours.

What is a.m. and p.m.? – a.m. is the time from the midnight to the noon (12:00 in extreme morning till 12:00 in afternoon) and p.m. is the period from noon to again midnight (12:00 in afternoon till 12:00 extreme morning/night).

Devices for time measurement

To measure time, we can use many devices, like – nowadays, use of analogue and digital clocks, (in the past, use of sundial and sand clocks). Let’s go in more detail of these devices.

1. Sundial

The ancient people did not had very good facilities, thus they measured time by the movements of sun, moon, stars and other heavenly bodies. Later on, the ancient Greeks had prepared a device which was the sundial. The sundial was having the intelligence which says that when the position of the rod placed on it changes means that time has also passed and accordingly they used to measure time.

2. Sand Clock

The second ancient device developed was the sand clock. The intelligence put in this device was when the sand in the upper chamber of the device falls in the lower chamber, a fixed amount of time has passed.

3. Analogue Clock

The third device which is a very common device for time measurement in the modern world. It’s the analogue clock. These clocks are in a circular shape (and sometimes other shapes) with numbers from 1-12 written on it in the circular manner. It has two hands or maybe three hands at times which can rotate from the centre point of the circular dial. The three hands have a specific size and thickness to indicate a specific time duration, like the second hand is the longest and thinnest which indicates the seconds. A little shorter and little thicker hand reads the minutes whereas the shortest and the thickest hand indicates the hours and accordingly we note down the time of the analogue clock. The 12-hour time has 60 minutes and the 60 minute scale has 60 seconds, if any.

4. Digital Clock

The fourth and the last device is the digital clock which as the name suggests, displays the time digitally in number format. It shows the time in either ‘hours and minutes’ or in ‘minutes, hours and seconds’.

Physical Quantities & Measurement | Physics | KnockLedge

Time Intervals

Now, we’ve talked a lot about time, let’s try to know about the time intervals. We use time intervals to measure the time of an event having a fixed duration. To get an appropriate duration of the event, we can start or stop the stop watches and stop clocks at the beginning and at the end of a task.

24-hour Clock System

In the 24-hour clock system, we use the number of hours that have passed since midnight. We do not repeat the numbers two times as in the analogue system. The time from 00:00 till 12:00 indicates the time duration from midnight to afternoon whereas, time from 12:00 till 24:00 (or 00:00) indicates time duration from afternoon to midnight.

E.g. – 02:30 hours means 2:30 a.m., 17:00 hours means 5:00 p.m.

(V.I.P Formula: 17:00 – 12:00 = 5:00).

Public facilities like railways and airlines have a non-stop 24-hour duty and thus they use the 24-hour clock system.

Main Features

The use of a.m. and p.m. in the timings is not there to avoid confusion.
We show the time by displaying 4 specific digits. The first two digits show the number of hours and the next two digits show the number of minutes.
We divide the hours and minutes by a colon (:) in 2 pairs of 2 digits.
Time continuously passes one number by number without any repetition.
The time 00:00 and 24:00 are both same.

Also read:

MEASURING AREA

The amount of surface enclosed within the boundaries of a plane (closed) figure is called its area. One square metre (m²) is the area of a square whose each side is 1 metre in length (1m 1m = 1m²).

The table below shows the formulas of finding area of some shapes:

Shape	Formula of area
SQUARE	Side × Side
RECTANGLE	Length × Breadth
TRIANGLE	1/2 × Base × Height
CIRCLE	π × r × r

How can you find the AREA OF IRREGULAR SHAPES?

Activity

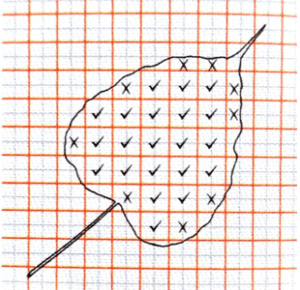
Aim: To measure the area of an irregularly-shaped leaf using a graph paper

Materials required: An irregular leaf, a graph paper and a pencil

Procedure: Place the leaf on the graph paper and trace its outline with the help of a pencil. Remove the leaf. Now count the number of complete squares within the outline and draw a ✔ on those boxes. Also count the number of incomplete squares that are half or more than half within the outline and draw a ❌ on those boxes. Ignore the number of squares that are less than half.

Observation: The area of the leaf

= (Number of complete squares) cm² + (Number of half or more-than-half squares) cm²

= 21 cm² + 9 cm² = 30 cm²

Conclusion: The area of an irregular shape can be measured by using a graph paper.

MEASURING VOLUME

When you fill a glass with juice, the juice occupies a certain amount of space in the glass. This space represents the volume of juice is the glass. The total space occupied by an object is called its volume.

MEASURING THE VOLUME OF SOLIDS

The volume of a solid figure is the total amount of space enclosed by it. Each unit of space in a solid figure is taken as a unit cube. A unit cube is a cube, the sides of which are of 1 unit in length. The volume of a unit cube is 1 cubic unit or unit volume. Imagine a brick made of number of unit cubes. So the volume of all the unit cubes together makes up the volume of the brick.

Instead of counting cubes, you can also apply a formula to calculate the volume of a regular solid. The given table shows the formula of volume of some regular solids:

Solid	Volume
CUBE	Side × Side × Side
CUBOID	Length × Breadth × Height
SPHERE	4/3 × π × r^3

UNITS OF VOLUME

The SI unit of length is metre. So, the SI unit of volume is: metre × metre × metre = metre^3 or m^3 (cubic metre). The volume of smaller solids is generally measured in (cubic centimetre). This is also written as cc.

1 m^3 = 1 m × 1 m × 1 m = 100 cm × 100 cm × 100 cm = 10,00,000 cm^3

MEASURING THE VOLUME OF LIQUIDS

Liquids do not have a fixed shape. They take the shape of the container they are kept in. The maximum volume of a liquid that a container can hold is known as its capacity. Capacity and volume have the same units.

The volume of a liquid is usually measured in litres (L) and millilitres (mL). It is measured using measuring cans that vary in shapes and sizes. These measuring cans are available from 50 mL to 5 L.

But, measuring cans have a specified value. Thus, they are used for commercial purposes and not for daily-life measurements. E.g. a measuring can having a measure of 1L cannot be used by a milkman to measure 200 mL milk. Precisely, these measuring cans do not have graduations (markings) on their outer wall.

In conclusion, we’ve explored the importance of ‘Physical Quantities and Measurement II’ in understanding our world. It’s like the language of science that helps us make sense of everything around us. Thank you, dear readers, for joining us on this journey. Your curiosity and interest in learning are truly appreciated. Keep measuring and discovering the wonders of our universe!

Thank you, dear readers for visiting our website theknockledge.com, and reading our article. Your support and interest mean a lot to us. I appreciate your time and hope you find value in my other articles too. Keep coming back for more knowledge and insights!